Main
-
Guides
-
How To: Compare Wind Speed vs Wind Power

How To: Compare Wind Speed vs Wind Power

Kinetic Energy

In order to quantify the power available in the wind we need to consider kinetic energy. Therefore, we need to understand how kinetic energy in atmospheric wind is calculated (see equation below).

$KE = \frac{1}{2}m v^{2}$

$m=mass$ $v=velocity$ $KE = Kinetic Energy$

The mass of wind

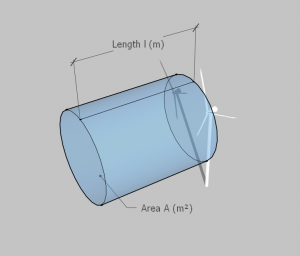
In order to calculate the mass of air colliding with the swept area of a wind turbine per unit time, consider the diagram below:

If we take A to equal the swept area of a particular wind turbine’s blades, and “l” as the length of the ‘tube’ of air that collides with the swept area per unit time, we can equate the mass of air to the following:

$m=A\times l \times\rho$

Where

$A=Swept Area$ $l = Length$ $\rho = density$

Length can be described in terms of velocity and time:

$l=v\times t$

Where:

$t=time$

Therefore if we substitute our new equations into the original equation for kinetic energy, we get the following:

$KE=\frac{1}{2}A\rho vtv^{2}$

If we combine the velocities we get:

$KE=\frac{1}{2}A\rho tv^{3}$

Power Available in Wind

Finally if we divide the above formula for kinetic energy by time, we end up with an equation for available Power. As shown in the equation below, the amount of power available is dependant on swept area, density of the air, and wind velocity.

$KE=\frac{1}{2}A\rho v^{3}$

Effect of Wind Speed on Available Power

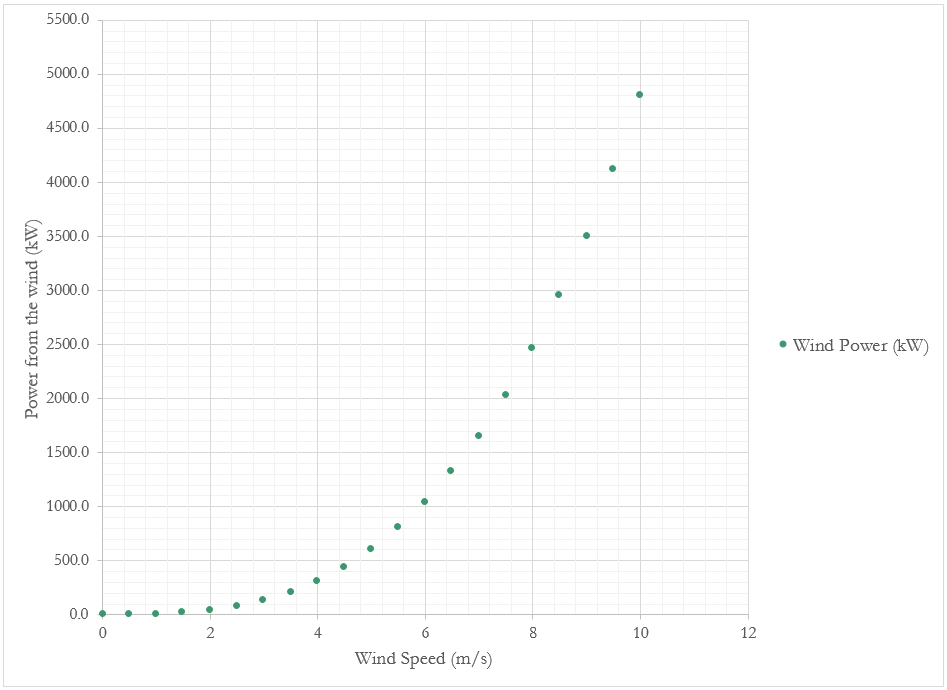
Now to the important part! If we plot the power in the wind against wind speed we get the following graph:

The relationship between wind speed and wind power is not linear! In fact, if the wind speed is doubled then the available power in the wind goes up by a factor of 8. This proves that accurately assessing the wind speed for a site is vital in terms of predicting electricity produced.